Theory reveals nature of SiC crystals defects
Polish researchers model crystals with complex defects such as edge dislocations
Imperfections of crystal structure, especially edge dislocations of an elongated nature, deeply modify basic properties of the entire material and, in consequence, drastically limit its applications. Using SiC as an example, physicists from Cracow and Warsaw have shown that even such computationally demanding defects can be successfully examined with atomic accuracy by means of a cleverly constructed, small in size, model.
This new model of SiC, built by physicists from the Institute of Nuclear Physics of the Polish Academy of Sciences (IFJ PAN) in Cracow, has allowed them to demonstrate that now it is possible to study crystals ab initio with such complex defects as edge dislocations and to explain their characteristics by processes occurring on an atomic scale.
This result, recently presented at the Multiscale Phenomena in Molecular Matter 2019 conference in Cracow, was achieved by the IFJ PAN physicists in cooperation with the Institute of Fundamental Technological Research of the Polish Academy of Sciences and the Institute of High Pressure Physics of the Polish Academy of Sciences, both located in Warsaw.
"We tried to find the mechanisms responsible at the atomic level for lowering the breakdown voltage in SiC crystals. Our ab initio calculations lead to a qualitative understanding of the problem and contribute to explaining the details of this phenomenon", says Jan Lazewski, professor at the IFJ PAN.
Ab initio calculations have now a long history related to Nobel Prize for Walter Kohn and John Pople in 1998 (however to linear crystal defect simulations they have only recently been introduced). This term is used to describe calculations carried out using quantum mechanics equations, supported only by knowledge about the structure of the atom and the symmetry of crystals. There is no direct information from experiments in such models, which means that they can also be used to analyse materials that have never been studied or even synthesised before. Because of relatively substantial complication of the issue, so far ab initio calculations worked, at most, in the case of point defects, related to vacancies (missing atoms or holes in the crystal structure) as well as admixtures introduced into the crystal.
It was not without reason that the Cracow researchers used SiC. Its band gap is almost three times greater than in silicon, the permissible conduction current density - twice as great, the ability to dissipate heat - more than three times greater, and the cutoff frequency of crystal operation as many as six times greater. In addition, SiC systems can operate at temperatures up to 650degC, while silicon systems already begin to have problems at 120degC. SiC also has a high melting point, it is hard, resistant to acid and radiation. Its disadvantages include above all the price: whilst two-inch silicon wafers cost only a few dollars, the value of similar SiC wafers runs into thousands. Low quality SiC crystals are a popular abrasive material, also used in bulletproof vests and in the brake discs of the world's most expensive cars, such as Lamborghini or Bugatti. High quality crystals are used to produce mirrors for telescopes and in high voltage devices with high resistance to temperature.
At the atomic level, SiC crystals are composed of many flat layers arranged one on top of each other. Each layer resembles a honeycomb: it consists of hexagonal cells in which the SiC molecules are located vertically in the corners. Each two adjacent layers can be combined in three ways. The multilayer 'sandwiches' with different layouts create so-called polytypes, of which there exist more than 250 in the case of SiC. The group from IFJ PAN used the 4H-SiC polymorph.
"When modelling such structures, one of the main problems is computational complexity. A model of pure crystal, devoid of admixtures or dislocations, is characterised by high symmetry and can be calculated even in a few minutes. In order to carry out a calculation for a material with dislocation, we need months working on a high power computer", emphasises Pawel Jochym, professor at the IFJ PAN.
The problems with edge dislocations result from the scale of their influence on the crystal structure of the material. As an illustration, they can be compared to the problem of disguising a gap in a row of tiles on a floor. The gap can be 'camouflaged' by moving the tiles of adjacent rows, but the defect will always remain visible. Edge dislocations resulting from the lack of whole lengths or regions of atoms/molecules in individual crystal layers act similarly, affecting the positions of atoms and molecules in many adjacent layers. And since the dislocations can extend over long distances, in practice the disturbances caused by them include the entire crystal.
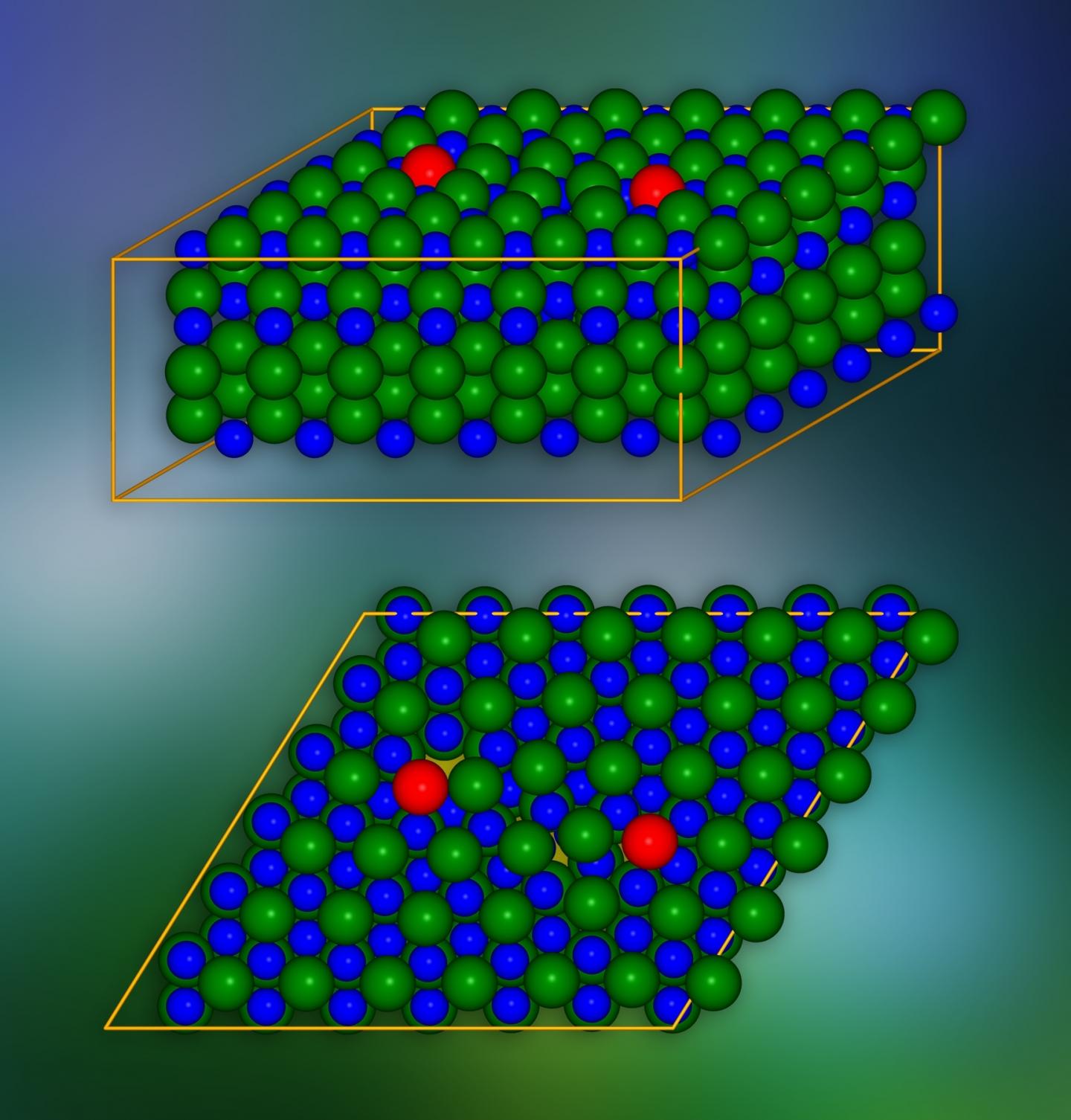
The most interesting phenomena take place in the dislocation core, i.e. in the vicinity of the edge of the damaged layer of the crystal network. In order to eliminate long-range effects caused by a single dislocation, and thus significantly reduce the number of atoms under consideration, a trick was employed: a second dislocation of the opposite effect was introduced. In this way, the impact of the first dislocation over longer distances was compensated for.
The SiC crystal model consisted of about 400 atoms. The simulations showed that in the layers of crystals, along the edge of the core of the defect, 'tunnels' appear in the form of channels with reduced charge density. They lower the potential barrier locally and cause electric charges to 'leak' from the valence band. In addition, in the forbidden gap, which in the insulator guarantees a lack of electrical conductivity, conditions appear which reduce its width and effectiveness in limiting the flow of charge. It was shown that these states originate from atoms located in the dislocation core.
"The situation can be compared to a deep, steep ravine that a squirrel is trying to cross. If the bottom of the ravine is empty, the squirrel will not get to the other side. However, if there are a number of trees at the bottom that are high enough, the squirrel can jump over their tops to the other side of the ravine. In the crystal we modelled, the squirrels are the electrical charges, the valence band is one edge of the ravine, the conduction band is the other, and the trees are the aforementioned states associated with the atoms of the dislocation core," says Lazewski.
Pictured above: SiC crystal model with edge dislocations introduced in places marked in red. A single crystallographic plane is presented at the bottom. The places where electric charges can 'leak' to neighbouring layers are marked in yellow.


































