LED DROOP - Physics beyond the common model

The LED is a flawed gem. On the plus side, it has a very long lifetime, an ability to reach full power in an instant and an efficiency exceeding that of conventional lighting sources. But on the downside its weaknesses include: a price that, while falling, is still too high for widespread adoption of solid-state lighting; and an output, in the case of the III-nitride devices that emit in the blue, green and white, that is severely limited by efficiency droop.
This malady, which is associated with a decline in efficiency at higher current densities, has been at the centre of a sometimes-fierce debate concerning the origin of droop, and possible remedies for it. Leading explanations for its cause include Auger recombination, carrier leakage, and density-activated defect-recombination.
Of these three, the mechanism receiving the greatest support is Auger recombination. This is a three-particle non-radiative process, where an electron and a hole recombine, transferring their energy to a third particle (an electron or a hole) as kinetic energy. It is established that Auger recombination takes place in III-nitride LEDs, because highly energetic particles have been observed in these devices. Collaboration between researchers at CNRS and UCSB enabled the observation of energetic electrons that are emitted through the surface of an LED chip, while optical experiments by a team at Osram Opto Semiconductors used quantum wells with different emission wavelengths to detect highly energetic electrons.
One of the two well-known alternative explanations for LED droop, carrier leakage, involves an increasing proportion of electrons lost to the p-side of the device at higher current densities. Despite the presence of an electron-blocking layer, it is argued that some of the electrons injected from the n-side of the p-n junction will spill over the active region and recombine with holes in the p-region, or even reach the p-contact. Several groups have reported evidence suggesting that carrier leakage may play a role in LED droop.
The third common conjecture for droop, density-activated defect recombination, is based on the idea that there is an energy barrier preventing carriers from reaching non-radiative defects. According to the model, this barrier decreases at higher carrier densities − and this enables the rate of defect recombination to increase superlinearly with carrier density.
There is no reason to believe that droop must be a result of just one of these mechanisms, and the other theories have no role to play in device behaviour. It is certainly plausible that all these energy-sapping mechanisms are at play in every device, making contributions that vary with the architecture of the LED and its material quality.
To analyse the dependence of efficiency on current density, many researchers investigating droop are using the so-calledABC model. This describes the recombination rate in a semiconductor with a combination of: a linear term in carrier density for Shockley-Read-Hall (defect) recombination, a quadratic term in carrier density for the radiative band-to-band recombination, and a cubic term in carrier density for band-to-band Auger recombination. The linear, quadratic and cubic terms have A, B and C coefficients, respectively, accounting for the name The ABC Model. It is based on a single-particle picture applied to a non-degenerate semiconductor, and it has been verified for a range of semiconductors with narrow to moderate bandgaps.
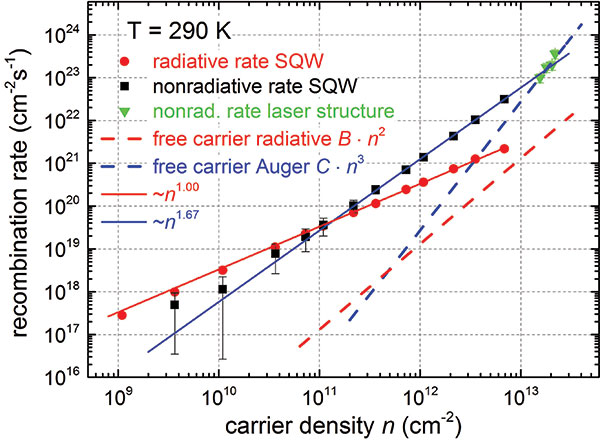
Figure 1: Comparison of experimental values (full symbols) for the radiative (red) and non-radiative (blue) recombination rates versus carrier density in GaInN/GaN quantum wells with the ABC model (dashed lines). At the highest densities, an Auger-like behaviour of the non-radiative rate is observed. At lower carrier densities, both radiative and non-radiative lifetimes behave non-classically, i.e. exhibit slopes of 1.0 and 1.67, contradicting the ABC model.
One of the downsides associated with using the ABC model to evaluate the LED is that it is not possible to directly measure the carrier density from either the quantum efficiency or the current density. Both of these quantities are functions of the recombination rates, such as of the sum of the A, B and C terms. This is a major drawback, because it means that measurements of LED efficiency as a function of current "“ the plots that are needed to gauge the extent of droop "“ are unable to determine the validity of applying the ABC model to the device.
The good news, however, is that it is possible to determine the relationships between carrier density and radiative and non-radiative recombination rates independently of each other with optical techniques. This is the approach that our team has pursued at Braunschweig University of Technology, where we have employed time-resolved optical spectroscopy to analyse recombination mechanisms [1]. This effort has involved using ultrashort laser pulses with variable pulse energy to resonantly excite InGaN quantum wells. The carrier density created by these pulses is directly proportional to the pulse energy, with the decay of the luminescence directly revealing the carrier lifetime at that given carrier density.
These experiments expose two fundamental flaws in the ABC model. One is that the radiative recombination rate in III-nitride quantum wells is not proportional to the square of the carrier density, as assumed in the ABC model: Instead, it depends linearly on carrier density. The second flaw is related to the non-radiative recombination rates at high carrier densities. They vary approximately quadratically with carrier density, rather than showing the cubic dependence that is expected for non-radiative Auger recombination rates in the classical picture.
Although these findings will surprise some of those investigating droop, they should not be that shocking. That's because as far back as the 1990s, deviations from the ABC model were observed in "˜good old' silicon. There, the Auger recombination rate at moderate carrier densities varies in a quadratic rather than cubic fashion [2,3]. Note that despite its indirect bandgap, Auger recombination is an important phenomenon in silicon − it is a major limiting factor on the quantum efficiency of solar cells made from this material [3].
The reason why the ABC model fails to capture the relationships between both radiative and non-radiative recombination and carrier density is that it does not consider the attractive Coulomb interaction between electrons and holes in the semiconductor.
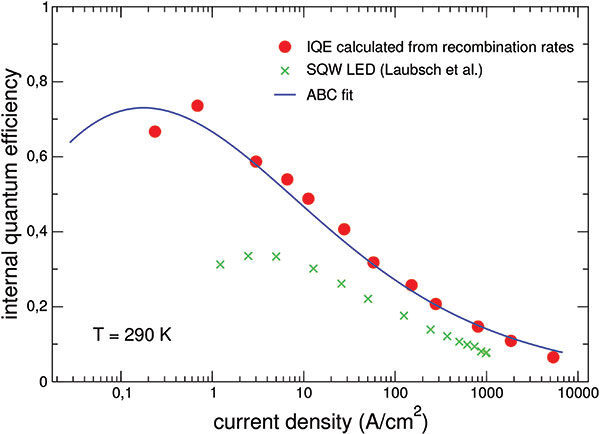
Figure 2: Calculated values of internal quantum efficiency as a function of current density, based on measured radiative and non-radiative recombination rates (full circles). The data are well fitted by the ABC model (full lines), even though the original dependence of the recombination rates contradicts the ABC model. For comparison, a typical LED droop curve (Laubsch et al. IEEE ED-57 79 (2010)) is shown (crosses), demonstrating very similar behaviour.
This interaction leads to the formation of a mobile bound state, like a hydrogen atom, that is known as a free exciton. When an electron and a hole are confined within an exciton, their spatial overlap increases significantly, and this leads to an enhanced radiative (and non-radiative) recombination probability. Due to this mechanism, the recombination rate depends on the density of excitons, which is governed by the carrier density.
A quantitative description of the radiative and non-radiative processes in semiconductor materials can be realised by modifying the ABC model with so-called excitonic enhancement factors that represent the density-dependent increase of the recombination probabilities [2,4]. In this more realistic model, excitonic enhancement factors are calculated from a many-particle theory that accounts for free-carrier screening of the Coulomb interaction and phase-space blocking.
The effect of excitonic enhancement on Auger recombination is even highly significant in silicon, a material with a bandgap below that of LEDs. In this material, the exciton binding energy is around 15 meV, about one half of the thermal energy at room temperature. In wide-gap III-nitride-based quantum wells, as used in blue LEDs, excitonic enhancement is far stronger, due to an exciton binding energy that can be as large as 50 meV, which is about twice the thermal energy. In this III-N, one would expect the effect of excitonic enhancement to be far greater − and it is, according to the strong deviations from the ABC model.
Do these considerations reveal anything about the cause of the droop? Absolutely. By taking data for the radiative and non-radiative recombination rates obtained from our optical experiments, we can calculate the efficiency of an LED at different current densities, generating a plot that shows a typical droop curve for this device (see Figure 2).
Note that even though our carrier-density-dependence of the recombination rates is quite different from that prescribed in the ABC model, our efficiency curve at differing current densities is indistinguishable from a real LED, demonstrating the ambiguity of typical droop analysis [5].
A further important conclusion from our studies is that an efficiency droop can be observed even under selective optical excitation, where carrier leakage does not occur. Consequently, this observed droop is merely related to recombination processes. Our measured non-radiative recombination lifetimes at high carrier densities are sample-independent and depend only weakly on temperature, but decrease towards high densities. This implies that an intrinsic (i.e. not related to crystal defects) non-radiative recombination process like Auger recombination must be dominant.
When we take into account the excitonic enhancement of Auger recombination, it follows that the recombination rate for this process varies like the square of the carrier density, rather than showing a cubic dependence. Consequently, Auger recombination constitutes at least the intrinsic limit of the droop phenomenon.
Our studies reveal that the widely used ABC model has major flaws that hamper studies of efficiency droop in III-nitride LEDs. To really understand the physics of droop, it is essential to account for the excitonic enhancement of both radiative and non-radiative recombination processes. Take this approach, and the dominant culprit for droop is unveiled as an intrinsic one: excitonic Auger recombination.
![]()















































.jpeg)













